
Statistical Field Theory and applications
This course is about how to describe complex systems using ideas of the renormalization group (‘coarse-graining’) and statistical field theory.
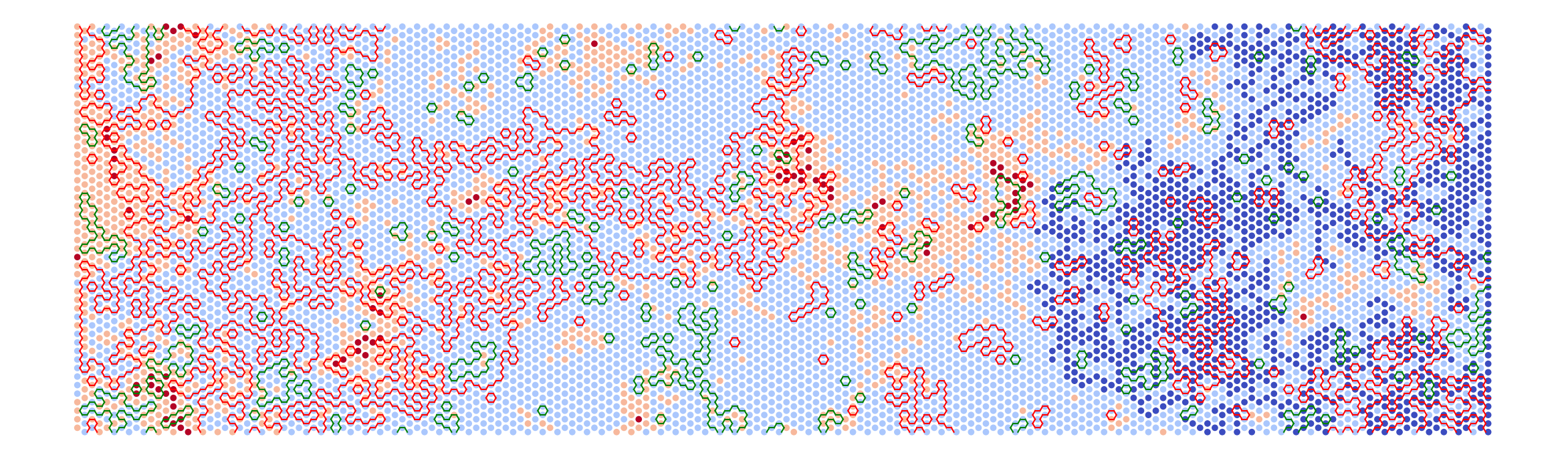
This course is about how to describe complex systems using ideas of the renormalization group (‘coarse-graining’) and statistical field theory. Taking lattice models of magnetism as a starting point, we will explore a few milestones of 20th century many-body physics, such as Wilson-Fisher fixed point and Kosterlitz-Thouless transition.
We will explore
— Examples of scale invariance
— Real space renormalization group
— Renormalization group formalism
— Applications of Landau Ginsburg theory and mean field
— Free field theory (as a conformally invariant fixed point)
— The large n limit in the O(n) model
— Wilson-Fisher 4-epsilon expansion
— Nonlinear sigma model and 2+epsilon expansion
— Field theory duality (XY model and Sine-Gordon)
— Kosterlitz Thouless RG flows
— The ‘landscape’ of the O(n) model in various dimensions
— Mapping between quantum and classical statistical mechanics
We will touch on further topics such as gauge theory and topological order if time allows.
Basic concepts from statistical mechanics such as partition function, phase transition, symmetry breaking. Familiarity with the idea of a functional (field) integral. Gaussian integration.
Written final exam and homework problem sets (3-4 submissions throughout the semester).
- John Cardy, Scaling and Renormalization in Statistical Physics, Cambridge University Press
- Denis Bernard, Statistical Field Theory and Applications, An Introduction for (and by) Amateurs https://www.phys.ens.fr/~dbernard/Documents/Teaching/Lectures_Stat_Field_Theory_vnew.pdf
- Mehran Kardar, Statistical Physics of Fields, Cambridge University Press